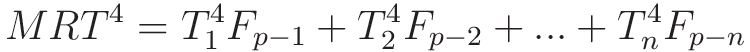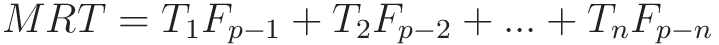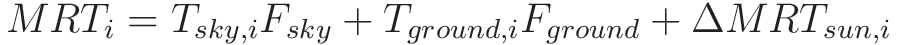Documentation
CFD
Tutorials
UI
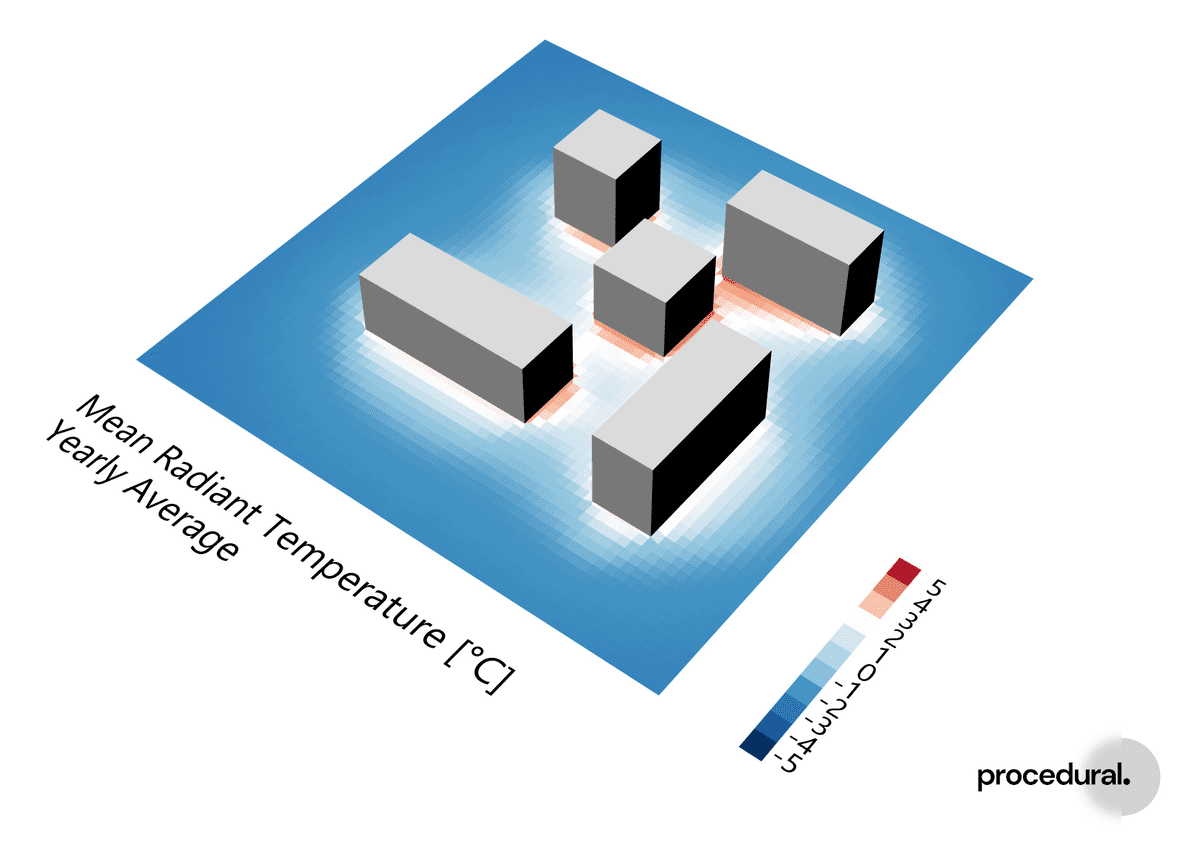
Mean Radiant Temperature
Definition
Mean radiant temperature (MRT) is a measure of the average temperature of the surfaces that surround a particular point, with which it will exchange thermal radiation.1
The amount of radiant heat lost or received by the human body is the sum of all radiant fluxes exchanged with the surrounding sources, MRT can therefore be calculated from the temperature of the surrounding surfaces and their positions with respect to the person.
Therefore, it is necessary to simulate those temperatures and calculate the angle factors between the person and the surrounding surfaces. Most building materials have a high emittance ε, so all surfaces in the room or outdoors can be assumed to be black. Because the sum of the angle factors is unity, the fourth power of MRT equals the mean value of the surrounding surface temperatures to the fourth power, weighted by the respective angle factor.2
Where:
- MRT is the mean radiant temperature in °C
- Tn is the temperature of surface n in °C
- Fp-1 is the angle factor between a person and a surface n
If relatively small temperature differences exist between the surfaces of the enclosure, the equation can be simplified to the following linear form
This linear formula tends to give a lower value of MRT, but in many cases the difference is small.
Outdoor Method
The MRT formula is rather straight forward to calculate indoors, but outdoors it becomes a little more complicated because we have to take the sky and sun into consideration. The method we use to calculate the MRT on Compute is coming from this paper
Where:
- MRTi is MRT for the hour i in °C
- Tsky, i is the sky temperature for the hour i in °C
- Tground, i is the ambient air temperature for the hour i in °C
- Fsky is the view factor to the sky
- Fground is the view factor to everything that is not the sky
- ΔMRTsun, i is the MRT contribution from the sun for the hour i in °C
By applying this formula to every face center of mesh we are able to generate a spatial map of the MRT for every hour of the year.
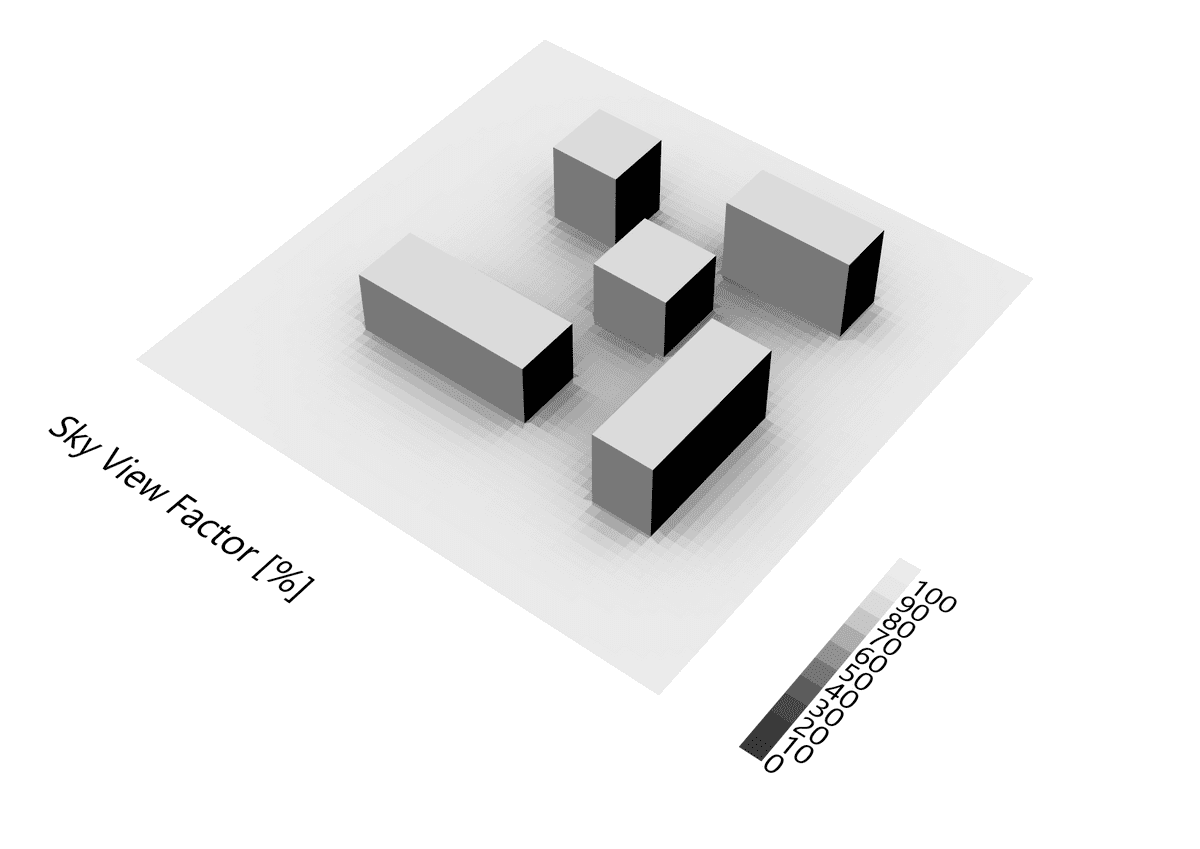
View Factor
Calculating the sky view factors can be done relatively easy with the help of Radiance. Compute already have capabilities for computing sky view factor as a standalone study, but running the MRT simulation we pack that simulation nicely with the other tasks.
The view factors are a value between 0 and 1, where 0 means that the analysis point cannot see the sky dome and 1 means that the point can see the complete sky dome. Since all view factors must add up to 1 for every point, we can simply subtract our sky view factor from 1 to get the ground view factor.
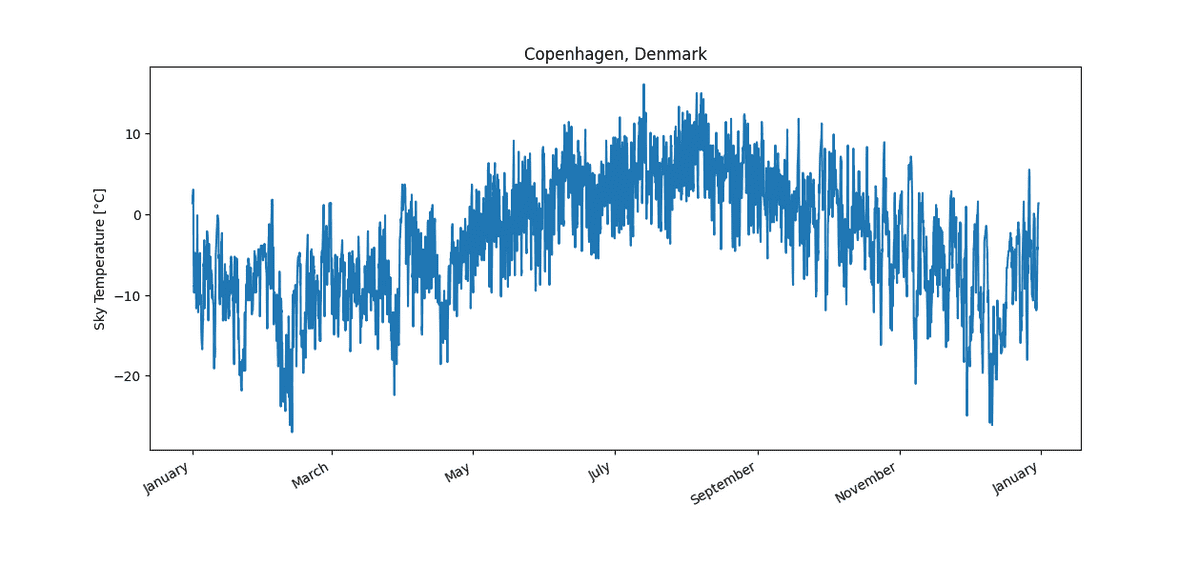
Sky Temperature
Using the same formula for sky temperature as EnergyPlus it is fairly easy to calculate the sky temperature for the whole year from an EPW file.
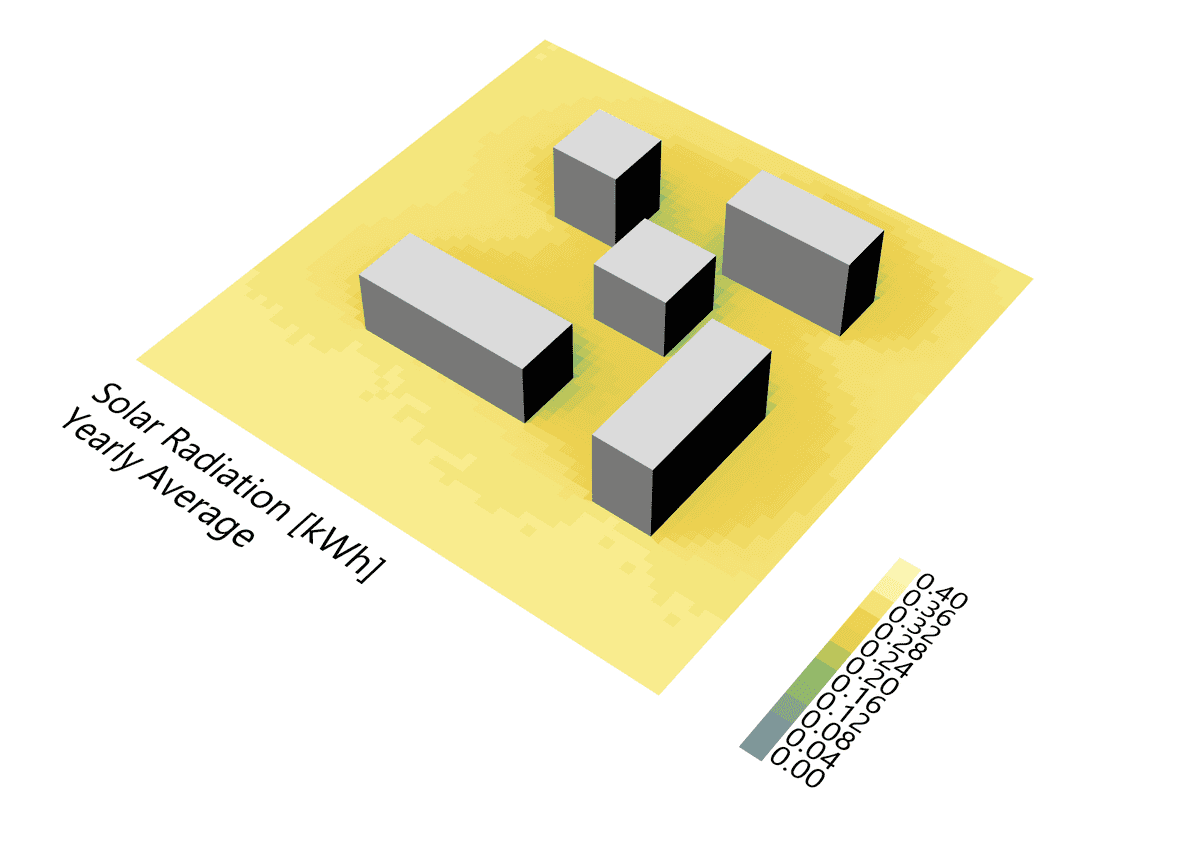
Solar Radiation
The ΔMRTsun, i component from the outdoor MRT formula above comes
from this paper
.

Where:
- ERF is the effective radiant field in W/m2
- feff is the fraction of body exposed to sun
- hr is the heat transfer coefficient in W/m2K
The ERF is dependent on the solar radiation as well as the view factors. We therefore, run a solar radiation simulation to obtain solar radiation values for each point in our mesh.
Mean Radiant Temperature
Finally, we can add the three contributions to the MRT up and generate the MRT for our project: